A (reverse) rational circle?
In this week’s This Week’s Find in Mathematical Physics (Week 286) Baez describes a construction of a rational homotopy circle. Thought it would be interesting to take a look at a the beginnings of the simplest “huge nightmarish space”. Edit: But as Josh clued me in, this isn’t exactly it.
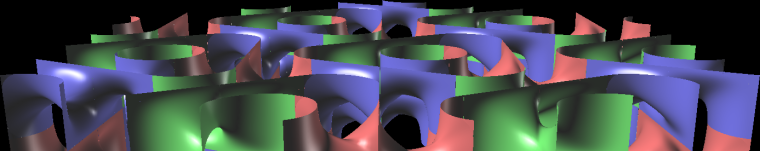
I’ll mainly just show some pictures and direct you to Baez’s post for details. His construction is just above the pirates. In red, orange, and green are the first, second, and third cylinders. This is an abstract space, so the particular immersions shown are rather irrelevant. It’s the attaching that matters. Edit: The attachings shown here are reversed from the ones Baez describes.

|

|

|

|
Here are a couple other different configurations.
There’s a few more in this set.
Would’ve done one more stage, but the quick way of doing it made my computer unhappy by the third stage and the not-so-quick way would’ve been a bit tedious in the fourth. Regardless, doubt it would’ve been enlightening. There’s surely a slicker way of doing it, but I didn’t think too much about it.
Edit:
Here are some newer pictures with the gluing maps going the correct direction. And perhaps it’s best to see the colored parts as cones of the gluings while the cylinders have been retracted to just black curves. Then the black curve on the free end of the red is the original circle, the red is the first gluing map, and the black curve between the red and orange is the first cylinder. And so on…
Seem to have gotten myself somewhat turned around and confused by this. Still a bit unsettled….
The original circle is homotopic to 2 times the curve between the red and orange and thus homotopic to 6 times the curve between the orange and green. So if the original circle is the loop g and n=3, then where’s the loop h such that g=3h?
Edit: One more pic to better show the progression of the construction.
Oh, and I found h.











Thank you,
some great pictures for that fantastic post of John.
I’m not seeing how this fits with John B’s explanation. Could you explain a bit more?
Ack! The perils of not thinking too much about it! Looks like I have the gluing maps backwards:
Walking around the top of the red walks twice around the bottom. Walking around the top the orange walks around three times its bottom, and six times the bottom of the red.
Thanks for pointing that out.
It’s funny – just like you drew it backwards, I *wrote* it backwards the first time I wrote it. But then I fixed it. The idea is that when you’re at the nth stage of building the telescope, marching once around the edge of the last cylinder you glued on is homotopic to marching n factorial times around the edge of the very first cylinder.
I’m too lazy to count if the free edge of the red cylinder in your latest picture spirals around 24 times, but it looks about right!
Would it be okay if I attach a copy of that picture to This Week’s Finds, crediting you and linking to this blog entry?
Yeah, the backwards one felt right when I was doing it. So I foolishly just ran with it without thinking. But that factorial property makes forward feel even better. In the now last pic you can see the 2x3x4 wrappings of the free red end with respect to the 1 wrapping of the free green end.
Thanks for the comments.
And by all means, please feel free to use any of the pictures.
(Hmm… For some reason it’s not replying to your earlier comment.)
I think I get it now. It’s funny because after the This Week’s Finds post I googled and googled for some pictures. I only had to be patient. What programs are you using for these great pictures, BTW?
These pictures are done using Rhino 3D. Actually I’m using the beta version of their port to OS X. There’s a function (called Flow) that lets you map a “spine” of an object to another curve to tell it how to deform the object. This is how I went from the chopped open version to the round one. It’s also how I managed to make the orange wrap around the green and the red wrap around the orange.
Some other posts are done using SketchUp.
Hey Ken,
There’s a great example of a Seifert fibered 3-manifold whose fundamental group is Q. This is in an introduction of a preprint by Geoff Mess. Take an infinite sequence of solid tori, and embed the nth torus into the n+1st by a cabling winding n times around. The union has fundamental group Q. I think this may be related to Baez’s example by thickening up.
neato!
Actually, I must have misremembered – the manifold is a graph manifold, not Seifert fibered. When you iterate a cabling, the Seifert fiberings don’t line up, of course. The graph structure is not canonical, either.